It
is a method of transmitting information using a carrier wave. The
frequency of the carrier wave is varied in accordance with the
amplitude and polarity of the input signal, the amplitude of the
carrier remaining unchanged
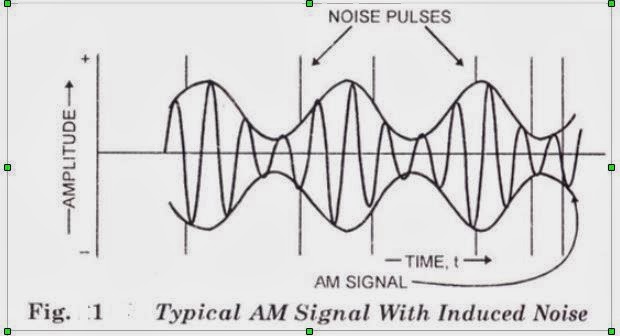
In ampli tude
modulation (AM) there is interference, such as static, lightning and
man made noise. These noises largely effects amplitude of RF wave.
This is because the noise is added and superimposed on the
transmitted AM signal. This increases the overall amplitude of the
signal as depicted in Fig. 1. That’s why we prefer frequency
modulation over amplitude modulation.
Modulated FM
Carrier:
When the FM carrier
is modulated, the frequency of the carrier varies by an amount that
is proportional to amplitude of the modulating waveform, this occurs
at a rate that is determined by the modulation frequency.
Fig. 2 graphically
illustrate what happens when a carrier is frequency modulated fig.2
(a) shows one period of an audio signal that will be used to
frequency modulate a carrier
Figure 2 (b)
illustrates how does the audio signal [shown in Fig. 2 (a)] affects
the carrier.
At time
t
= <0,
RF carrier operating at a specific frequency
t=0,the
modulating signal is applied to the RF carrier.
t=1
, the frequency of the modulated RF carrier has increased
proportionally.
t=2,
the amplitude of the modulating signal attains a maximum value. At
the same time, the RF carrier has increased to its maximum frequency.
t=3,
the modulating waveform begins to decrease in amplitude and the
carrier frequency also begins to decrease [Fig. 2 (b)].
t=4,
the modulating wave returns to zero and the carrier frequency returns
to the resting frequency.
t=6,
the modulating signal has decreased to its maximum negative value and
the frequency of modulated carrier also reaches its minimum [Fig. 2
(b)].
t=7, the
modulation amplitude begins its return journey back to zero
t=8,
the carrier frequency returns to the resting frequency.
Observations:
• As the amplitude
of the modulating source increases, the frequency of the carrier
increases.
• As the amplitude
of the modulating source decreases, the frequency of the carrier
decreases.
• The amplitude of
the FM modulated carrier remains constant as the amplitude of the
modulating source varies.
Table 1 illustrates
how a modulating signal affects the FM and AM modulated waveform.
TABLE 1.
Modulation
Effects on AM Vs FM
Modulating
Increases
|
Amplitude
Decreases
|
|
FM
|
Frequency swing
increases
|
Frequency swing
decreases
|
AM
|
Carrier level
increases
|
Carrier level
decreases
|
Power Relation
in FM Carrier Wave:
The amplitude of the
carrier wave is not affected during the process of frequency
modulation. Hence the total power in the wave remains unchanged
irrespective of the degree of modulation. In frequency modulation,
only the capacitance of the oscillator tank circuit is changed due to
the modulating signal so that a change in frequency of oscillator
takes place but the power output of the oscillator remains
unaffected during the process of frequency modulation.
Analysis of
FM
Carrier
Wave:
Let the carrier and
modulating voltage waves be represented as
vc
= Vccos
(wct
+
ϴ) and vm
= Vmcos
(wmt)
where , Vc,
wc
and ϴ are the instantaneous value, peak value, angular velocity and
the initial phase angle of the carrier and vm,
Vm
and wm
are the instanta neous value, peak value and the angular velocity of
the modulating signal.
Let øc
(t) = wc
t + ϴ(f)
is
the total instantaneous phase angle of the carrier wave at time t.
vc
= Vccosøc(t)
...(1)
The instantaneous
angular velocity wc,
defined as the instantaneous rate of increase of instantaneous phase
(or angular displacement), is related to phase angle øc
as below
wc
= dø/dt ...(2)
In frequency
modulation, the frequency of the carrier wave varies with time in
accordance with the instantaneous value of the modulating voltage.
Thus the frequency of the carrier after frequency modula tion is
given as
w= wc
+ Kfvm
= wc
+ KfVmcoswmt...(3)
where K, is a
constant of proportionality
The total
instantaneous phase of the FM wave is obtained by integrating Eq. (3)
i.e.,
ø(t)
= ∫wdt
=∫(wc
+ KfVmcoswmt)dt
= wct
+ KfVm
(1/wm
sin wmt
+ ϴ1)
Where ϴ1 is the
constant of integration and represents the initial phase. The initial
phase ϴ1 may be neglected since it is insignificant in the
modulation process. So we have
ø(t)
=
wct
+ KfVm
/w msin
w mt
...(4)
The equation of FM
wave is given by
vfm=
Vcsinø(t)
= Vc
sin ( wct
+ KfVm/wmsinwmt
)....(5)
The instantaneous
frequency of FM wave can be
had from Eq. (3) i.e.
f=w/2π=wc/2π+kfVm/2π
coswmt
=fc
+kfVm/2π
coswmt
From above Eq. the
maximum and mini mum values of frequency are given as
fmax
=fc
+kfVm/2π
fmin
=fc
-fd
Thus the frequency
deviation,
defined
as the maximum change in frequency from mean value fc,
is
given as
fd
=fmax
-fc=fc
-fmin
=kfVm/2π
The total variation
in frequency from the mini mum to maximum value is
called the carrier
swing and
it is given as
Carrier swing, CS =
fd
=
kfVm/π
The frequency
modulation index mf,
defined as the ratio of frequency deviation to modulation frequency
(i-e.
fd/fm),
is
given as
mf
=
fd
/
fm
=wd
/wm=kfVm/wm
Thus the equation
for the FM wave [Eq. (5)] in terms of mf,
becomes
vfm=
Vcsin
[wct
+ mfsin
wmt]
or
ufm=
Vc
[sin wctcos
(mm
sin wm
t) +
coswc
t sin (mf
sin
wm
t] ...(6)
Percent of
Modulation in FM:
In AM,100%
modulation is defined as the point where the amplitude of the RF
carrier rises to twice the nor mal amplitude at its maximum and drops
to zero at its minimum. Anything greater than 100% modula tion, in AM
causes distortion to the modulated wave.
With FM, it is the
amount of frequency deviation that determines the degree of
modulation. Actually the main difference between amplitude and
frequency modulation is that in amplitude modulation the modulation
index can be max 100% but in frequency modulation it could be greater
than 100% and even no information is clipped off.
Modulation Index:
Modulation index is
defined as the ratio of the frequency deviation to the frequency of
the modulating signal.
Frequency
modulation index,
mf
=
kfVm/wm
From above equation
it is obvious that for a given frequency deviation or for a constant
amplitude of modulating voltage, the modulation index varies
inversely as the modulating frequency fm.
Unlike amplitude
modulation, the frequency modu lation index can exceed unity.
FM DETECTION
For the detection of
FM waves, the method usually employed involves the conversion of FM
into AM and then application of conventional methods of detection.
Thus, demodulation of an FM wave involves three operations which are
given below :
(i) Conversion
of frequency variations produced by modulating signal into
corresponding am plitude variations.
(ii)
Rectification
of modulating signal
(iii)
Elimination
of RF component of the modulated wave.
For the FM detection
we need a circuit in which magnitude of output voltage varies in
accordance with the instantaneous frequency variation in the input
voltage. Such circuits are called discriminators.
Slope Detector:
The circuit diagram
of a slope detector is depicted in Fig. 3. The circuit uses two tuned
circuits which are tuned to two different frequencies. First one is
tuned to the incoming FM carrier frequency wc
where
as the second is tuned to a frequency slightly different from the
carrier frequency. Thus, this portion of circuit which contains two
tuned circuits tuned to different frequencies, is called
discriminator. This circuit converts FM into an AM signal as shown in
the slope detector characteristic curve. The another portion of the
circuit is envelope detector. The AM signal from the output of the
discriminator is applied at the input of the envelope detector. At
the output of the envelope detector, the original modulating signal
is obtained.
Shortcomings:-
- The circuit's nonlinear characteristic produces a harmonic distortion.
- The circuit does not eliminate the amplitude vari ations and the output is sensitive to any ampli tude variations in the input FM signal which is obviously not a desirable feature
- It is less efficient.



No comments:
Post a Comment
Note: only a member of this blog may post a comment.